Reflection #1: Unit Q

#1. What does it actually mean to verify a trig identity?

You show that the Pythagorean Theorem is correctly used in the triangles to find sine and co-secant, cosine and secant, and tangent and cotangent. For example,  if you are given sine=3/5 you know that cosine must equal 4/5. You get the 4 from the Pythagorean Theorem 3^2+b^2=5^2; b=4. Meanwhile, you show that you get the the 5 as the denominator as well as the c value in the Pythagorean Theorem because we know that sine is S-O-H, opposite/hypotenuse and cosine is C-A-H, adjacent/hypotenuse. Also, tangent is T-O-A, opposite/adjacent. ( Soh cah toa is use for right triangles only).

To better visualize the concept, please take a look at the images below:





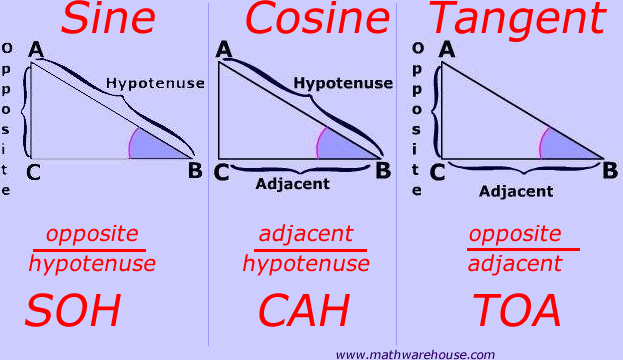
http://www.mathwarehouse.com/trigonometry/images/sohcohtoa/sohcahtoa-all.png





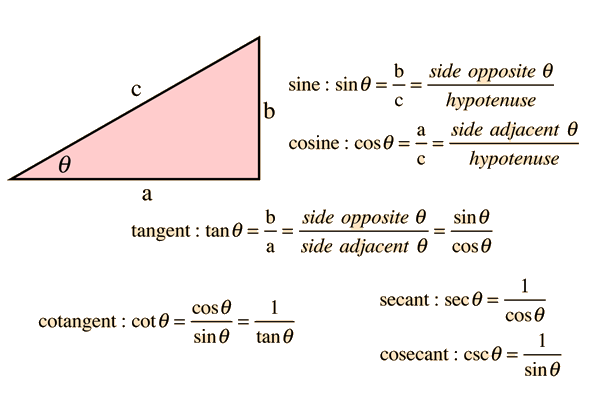
http://hyperphysics.phy-astr.gsu.edu/hbase/imgmth/ttrig.gif



#2. What tricks and tips have you found helpful?

I found that knowing the trig identities out of the top of your head was really helpful. However, there is no shame in not being able to memorize them quick enough or at all. I do think is better if you do know them because then you are more familiar with the concept and you have a better idea of how to get where you want to get. I suggest you do Mrs. Kirch's blog posts with thoughtfulness and to practice as much as possible and to ask questions. I also remember having problems in which knowing how to factor pretty good will help you a lot in simplifying or verifying.





#3. Explain your thought process and steps you take in verifying a trig function. Do not speak in specific manners, but speak in general terms of what you would do no matter what they give you.

My thought process would be:

• can I substitute an identity?
• can I use ZPP?
• are the numbers in the problem familiar with unit circle numbers?
• do I have to Square anything?
• does it deal with fractions?

1. - 

do I know how to deal with fractions(ex. when you multiply a fraction you do not have to have like terms!)